Definition: Beer-Lambert Law is the linear relationship between absorbance of electromagnetic radiation and the concentration of an absorber in the medium through which the radiation travels. The general Beer-Lambert Law would be: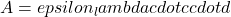
where A is the measured absorbance (i.e.
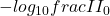, I is the intensity of the measured light and
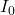is the intensity of the incident light),
is the wavelength-dependent molar (decadic) absorption coefficient (formerly molar decadic extinction coefficient) with units of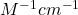
, c is the concentration of the absorber (
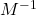) and d is the pathlength of light (cm). Please note that the subscript
is often dropped with the understanding that the value for
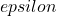is for a specific wavelength.
Alternative definition:
Synonym: Beer-Lambert-Bouguer Law
References: https://doi.org/10.1002/cphc.202000464
https://doi.org/10.1017/CBO9781139029797
Related terms: modified Beer Lambert law, optical density, molar absorption coefficient
